Home > Features >

QFI Archives

IN QUEST OF INFINITY – 20

By Prof. G. Venkataraman

---

Loving Sai Ram and greetings from Prashanti Nilayam. I hope I have not lost you after I made the change of gear that took you from the Cosmos to Basic Physics. Just to remind you, I first offered a lightning glimpse of Physics as it evolved under Newton and later physicists [almost every one of them from Europe], till the end of the nineteenth Century. The highpoint of this period was Maxwell’s great Electromagnetic Theory of Radiation, into which all phenomena of optics could be neatly fitted. Indeed, Maxwell’s theory led to incredible practical benefits that are being conferred upon us right to this day; take anything from cell phones to microwave ovens, and we have to thank Maxwell for all of them.

You might say: “OK, but what has all that got to do with our Quest for Infinity?” I am coming to that but would have to do it in stages, for now the ball game gets rather subtle; and we have first to go through the rather mysterious subject called Quantum Mechanics. Right now, I am trying to set the stage for describing its birth! Hope all that reassures you that I have really not lost my way!

19 th Century Scientists Struggle with some Imponderables

The 'mini solar system' of the atom developed by Niels Bohr

Before I get to what I want to say this time, let us remember that Niels Bohr [see QFI 18] wanted to have a theory for why atoms were emitting the characteristic line spectra. To Bohr, it all seemed as if each atomic species was singing its own song, and he wanted to know why they were singing “songs” [read emitting spectra] in the first place, and why these songs [read spectra] were different from each other.

I told you that Bohr came up with a model based on the idea that an atom was really like a mini solar system with a tiny, tiny nucleus at the centre [that played the role of the sun] while the electrons moved around like planets, revolving around the nucleus in various orbits. Bohr tried to apply this idea to the hydrogen atom because it was the simplest among the atoms with only one electron going round the nucleus, which, by the way, was nothing more than a single proton.

The model was utterly simple but the result that Bohr got was disastrous. Bohr applied Classical Mechanics, which was all that one had at that time. And when Bohr went through his calculation, he found to his horror that the atom hardly lived, in fact less than a second. Imagine that! Here we are in a Universe that is billions of years old with hydrogen atoms all over the place. We have them here on planet Earth too. In fact, chemistry students are taught how to make gaseous hydrogen with what is called the Kipp’s apparatus. But Bohr’s theory said that the hydrogen atom had no business to exist! The electron must rapidly spiral into the proton, wiping out the hydrogen atom! Bohr knew that there was something wrong, and very correctly he suspected that the problem lay in the Classical Mechanics that he had applied to the hydrogen atom.

This is what I am trying to slowly draw your attention to. You see, even as the nineteenth century was drawing to a close and physicists were exuberantly celebrating the great triumphs scored during the nineteenth century, they were also getting signals that all was not quite OK with Classical Physics. The signals came from different directions, and often they did not seem connected. For example, there was Planck who found that a classical formula could not explain the spectrum of Blackbody Radiation [see QFI 19]. Then there was the photo-electric effect that defied explanation within the framework of Classical Physics. Meanwhile, J. J. Thomson discovered the electron; where did that come from? Becquerel discovered radioactivity; where did that come from? Röntgen discovered x-rays; where did those come from? It looked as if even as a new century was being ushered in, Nature was slowly raising the curtain on a whole new world of Physics! Some of that story we shall catch up with as we go along with our quest – by the way, that part of the story is very important to us!

Henri Becquerel		Wilhelm Conrad Röntgen

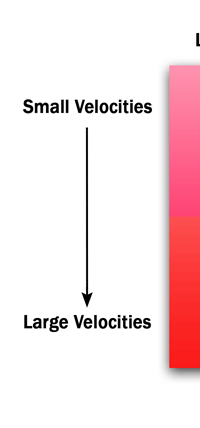
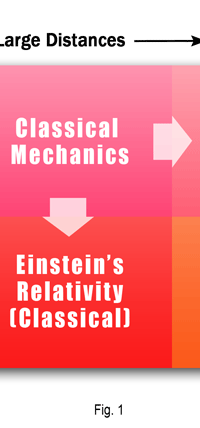
Moving at the Speed of Light

Let me now make the point I have been slowly inching towards all this while. You see, as the twentieth century began, Physics slowly began to cross two new frontiers. Frontier One was associated with very high velocities; what is meant by high? By high, I mean speeds approaching close to that of light. Remember, light travels roughly at 300,000 km per second, and just to give you an idea of what that means, let me tell you that it takes light eight minutes to reach us from the moment it leaves the Sun. If you think that is long, let me tell you that if a spacecraft close to say Neptune were to send an electronic signal now, it would take hours for that signal to reach us - this in spite of the signal travelling at the speed of light! Imagine having a telephone conversation with an astronaut on that spacecraft! You say hello, and that message takes several hours to reach him; he asks ‘how are you?’, and that takes more hours to reach the earth! To get back to what I was trying to say, we could make a diagram as below:

FIGURE 1: This schematic shows that while it all began with exploration of physical phenomena on large scales and concerning objects moving at slow speeds, the dawn of the 20th century saw a strong drift towards exploration of matter on small scales of length on the one hand, and of phenomena involving very high velocities on the other. The former gave birth to Quantum Mechanics while the latter gave birth to Relativity. Interestingly, while Quantum Mechanics broke entirely new ground, Relativity continued to rest on a classical foundation. What happens when one has to deal with very high velocities in the world of the small? That is when one has to invoke Relativistic Quantum Mechanics. Here QM is non-traditional while Relativity still maintains its classical roots. Currently, we are on the verge of exploring distances so small that Relativity itself may have to be modified to have quantum roots. At present, no one knows how exactly to do it, but most people are agreed that one day, both these revolutionary disciplines would have to come together in some manner or the other, perhaps each of them giving up a bit for the sake of the merger!

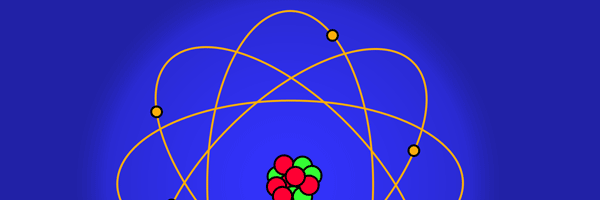
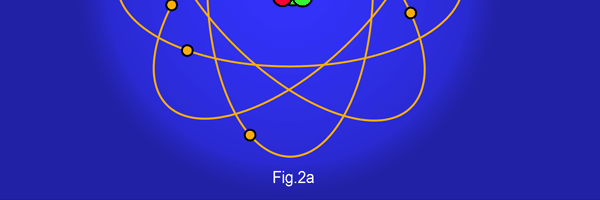
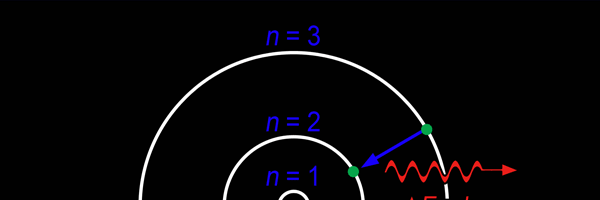
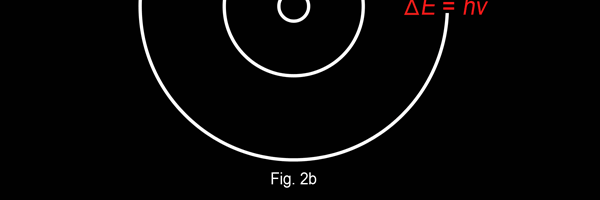
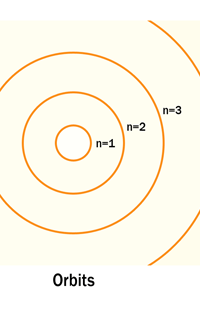
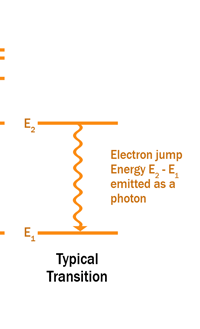
The above diagram is merely to put in perspective the developments that began regularly to shake Physics from about 1900 onwards; the story is not over, not by any means, and the most exciting chapter is yet to be written! Meanwhile, let us go back about a hundred years, when exciting events had just begun to happen. The year was 1912. Bohr tried a classical model for the hydrogen atom and failed. He knew something was wrong, but what was wrong and where? Where to put the finger to get at the solution? Bohr thought hard, and came up with a very original thought.

Bohr said: “Let us go back to Planck’s explanation for the blackbody radiation spectrum. There are two interesting aspects to his analysis. First is that the energy of mechanical oscillators is quantised [see Fig. 8 in QFI 19]. Next, we must remember that according to Planck, radiation energy is itself quantised. These two facts seem to indicate that when we enter the world of the small, quantum effects apparently enter in some mysterious way and simply cannot be ignored. My classical model for the hydrogen atom failed to deliver because it totally ignored all quantum effects. Maybe, I should build in quantum effects in some manner or the other.”

Starting thus, Bohr was able to tinker with his model and quickly come up with a brilliant improvisation. It was quite simple really, so simple that these days the Bohr model is taught even to high school students. So what was the great magic that Bohr performed? After a lot of thinking, Bohr argued, “When Planck tried to explain blackbody radiation, he assumed that the energy of the mechanical oscillators [remember the electrons on the walls of the cavity that were oscillating back and forth? See QFI 19] were quantised. This means that when one goes to very small distances, even mechanics begins to acquire some sort of quantum character. So why don’t I suppose that when an electron moves in an orbit around the nucleus, the angular momentum of the electron is quantised?”

Atomic Equations

For the benefit of those of you who do not know, I should mention that when an object of mass m moves with a velocity v, we say it has a liner momentum mv. Similarly, when an object of mass m moves in a circular orbit of radius r with a velocity v, then it has an angular momentum m v r. Now what Bohr was essentially saying was that the value of the quantity m v r could not be any arbitrary number but has to be one of a fixed set. What was that set? Here, Bohr made an inspired guess: “He said that Nature’s rule is that the angular momentum has to be an integer times the basic constant given to us by Planck, namely h.” Stated simply, Bohr’s rule was:

Angular momentum = (nh) / 2π,

where n is an integer and takes on values 1, 2, 3, …..etc. Now if you take this rule literally pulled out of a hat by Bohr, and remember that angular momentum is given by m v r, and further that m the mass of the electron is fixed, then clearly, only certain orbits would be allowed and not all the ones we might imagine to be possible.

Thus, Bohr took a bold step and said: “Nature has ordained, that of all the orbits that might be possible in the classical picture, in reality only a set of preferred orbits [that obey the angular momentum quantisation rule] are allowed. The electron can move in these special orbits without the danger of spiralling down; these orbits are divinely ordained and therefore safe! These are stationary orbits, and what saves the electron from destruction when it moves in these orbits is the fact that they conform to the rule of quantisation of angular momentum [given above].”

OK, all this is fine. But what about the light the atom emits? How does that come about in this picture? Bohr said that was no problem. And to understand Bohr’s explanation, take a look at Figure 2 below:

FIGURE 2: This figure has two parts. One of which (a) shows schematically, electrons orbiting around the atomic nucleus. The second part (b) is more indicative of what the Bohr atom model is all about. Here we see various orbits, each corresponding to particular value for n [remember, angular momentum must be n times Planck’s constant h, divided by 2π]. Orbits with low n values correspond to states of lower energies while those with higher n values correspond to states of the atom having higher energy. Bohr said that when an electron jumps from an orbit of a higher n value to an orbit of a lower n value, the energy difference is emitted as electromagnetic radiation. Thus, when the electron is in the state n = 3, its energy is higher compared to what it would have if n = 1. The state n = 1 is called the ground state, while states with n > 1 are called excited states. When the electron jumps from a state with n > 1 to say the state n = 1, we say the atom makes a transition from an excited state to the ground state, and the difference in energy is emitted as electromagnetic radiation with a particular frequency.

So you see, by introducing the idea of quantisation of angular momentum, Bohr neatly brought the atom into the fold of the quantum world. And from that, explaining line spectra was no sweat at all. First, take a look at the figure below.

FIGURE 3 This figure shows [schematically] not only the various allowed orbits of the hydrogen atom but also the associated energy levels [see the sketch on the right]. Light is emitted when the electron makes a jump from an orbit with a higher energy to an orbit with a lower energy. The frequency v of the emitted light is given by the simple formula: hν = E2 – E1, where E2 and E1 denote respectively the energies of the higher and the lower levels.

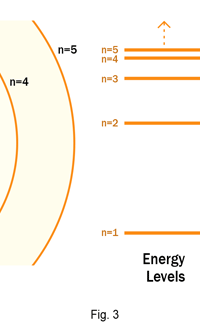
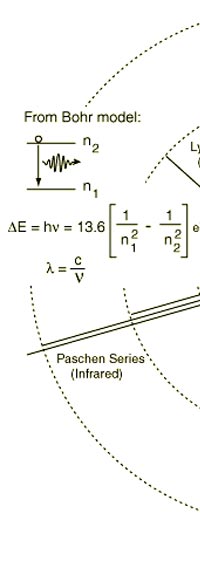
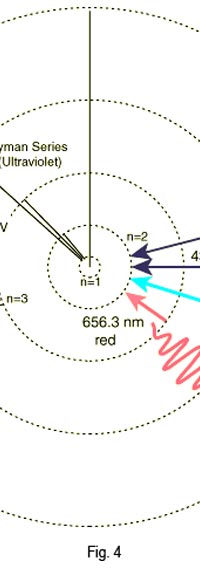
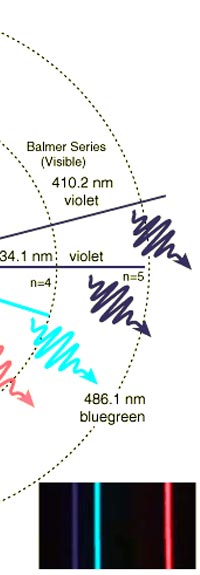
As they say, the proof of the pudding is in the eating of it. So how good was Bohr’s model of the hydrogen atom? It turned to be amazingly good! You see, physicists had already made a fairly detailed study of the spectrum of the hydrogen atom and found that the spectral lines could be divided neatly into various “families” known as the Balmer series, Lyman series, Paschen series etc., each series being named after the discoverer. Bohr showed that each series was the result of appropriate jump of the electron from higher energy levels to a particular lower energy level. Thus, as you would find from Figure 4.

FIGURE 4. This figure illustrates how the Bohr model of the hydrogen atom helps to understand the origin of the various families identified earlier in the atomic spectrum of hydrogen. The so-called Lyman series of lines are those that involve a jump of the electron from a state of higher energy to the lowest state, namely that with n = 1. These lines occur in the ultraviolet. Next come the Balmer series, which arise when the electron makes a transition from a higher state to the state with n = 2. These lines occur in the visible part of the spectrum. After this come the Paschen series, where the final state of the electron corresponds to the energy level with n = 3, and so on. Before Bohr, people knew there were all these lines but no one understood how they arose. Bohr’s model made it all so very simple!

Bohr’s model was unquestionably a great step forward. However, very soon, after all the cheering was over, people began to realise that Bohr’s model was just a model and not a theory. A theory is a regular grammar that lays down some basic rules which could then be applied to various situations. On the other hand, the model that Bohr gave was applicable only to the hydrogen atom. Indeed, as more careful experiments came to be done, it was found that it could not even explain everything about the spectrum of hydrogen, especially the finer details. Even worse, it was almost impossible to extend the model to the next simplest atom, namely helium, which had two electrons instead of one. If it was going to be so tough even for helium then what to say of more complex atoms like oxygen and so forth?

All this did not mean that people began to dismiss Bohr’s work. On the other hand, they realised that it was trying to give a coded message. Part of the message was clear, which was that if one wanted to understand atoms, then one had better be ready to apply quantum ideas. But how exactly was this to be done? No one knew, indeed for nearly fifteen years. After that incubation period when ideas were being cooked, the answers came, and they were of a quite surprising nature; indeed, they did not come because people were looking at more complex models for the atom. Rather, they came because physicists were now looking for an honest to goodness quantum theory. That story is reserved for the next issue!

(to be continued...)

	BIOGRAPHY: NIELS BOHR Niels Bohr   Niels Henrik David Bohr was born in Copenhagen on October 7, 1885, as the son of Christian Bohr, Professor of Physiology at Copenhagen University, and his wife Ellen, née Adler. His father was an eminent physiologist and was largely responsible for awakening his interest in physics while still at school, his mother came from a family distinguished in the field of education. After matriculation in 1903, Niels Bohr entered Copenhagen University. He took his Master's degree in Physics in 1909 and his Doctor's degree in 1911. While still a student, he did some research work in his father's laboratory for which he won a gold medal. The study was published in the Transactions of the Royal Society, 1908. Bohr then went to England, where he first spent some time in Canvendish, then headed by Sir J. J. Thomson, the discoverer of the electron. Later he moved to Manchester, to work in the lab of Prof. Rutherford who had discovered the atomic nucleus. It was there that he discovered in 1913, the atom model for which he subsequently became famous. In 1922, Bohr received the Nobel Prize for Physics. In 1920, he became the head of the Institute for Theoretical Physics in Copenhagen, a post he occupied till his death in 1962. Form 1930 onwards, Bohr became more interested in nuclear physics and made many contributions to that field. Incidentally, his son Aage Bohr, later won the Nobel Prize for the contributions he made to Nuclear Physics, in the very Institute that his father headed for a long time. Bohr also contributed a lot to the understanding of the philosophy underlying quantum mechanics, a topic to which we shall turn later. During the Nazi occupation of Denmark in World War II, Bohr escaped to Sweden and spent the last two years of the war in England and America, where he became associated with the Atomic Energy Project. In his later years, he devoted his work to the peaceful application of atomic physics and to political problems arising from the development of atomic weapons. In particular, he advocated a development towards full openness between nations. During the last years of his life, Bohr showed a keen interest in molecular biology.

Previous Articles In The Same Series
Part - 01	Part - 02	Part - 03	Part - 04
Part - 05	Part - 06	Part - 07	Part - 08
Part - 09	Part - 10	Part - 11	Part - 12
Part - 13	Part - 14	Part - 15	Part - 16
Part - 17	Part - 18		Part - 19

---